4.3.A
Let
be a non-singular dividing curve of
degree
. Let
,
be real lines,
be one of two
components of
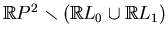
. Let
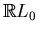
and
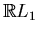
be oriented so that the projection
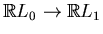
from
a point lying in
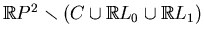
preserves the orientations. Let ovals
,
of
lie in

and
is
tangent to

at one point
. If the intersection
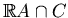
consists of
components, each of which is an arc connecting
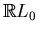
with
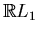
, then points of tangency of
with
and
with
are positive with respect to one of the complex
orientations of
.
Proof.
Assume the contrary: suppose that with respect to a
complex orientation of
the tangency of
with
is
positive and the tangency of
with
is negative. Rotate
and
around the point
in the directions
out of
by small angles in such a way that each of
the lines
and

obtained intersects transversally
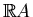
in
points. Perturb the union
and
obeying the
orientations. By
2.3.A, the nonsingular curves
and
obtained are of type I. It is easy
to see that their complex schemes can be obtained one from another by
relocating the oval, appeared from
(see Figure
28). This
operation changes one of the numbers
and
by 1. Therefore the left hand side of the complex orientation formula
is changed. It means that the complex schemes both of
and
can not satisfy the complex orientation formula.
This proves that the assumption is not true.
